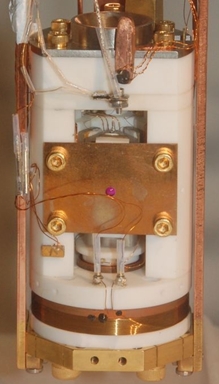
Scanning Tunneling Microscopy

Fig. 1 Photograph of the STM built in the Hoffman lab.

Researchers

Tess Williams, Anjan Soumyanarayanan, Michael Yee

Instrument

• Simple explanation of STM (no equations)
• Technical explanation of STM
• Measurements we can make with an STM

We have constructed a custom low temperature scanning tunneling microscope with the following features optimized for the study of cleavable correlated electron materials:

• Surface preparation: In-situ, low temperature sample cleaver for exposing a clean sample surface in a cryogenic ultra-high vacuum (UHV) environment.
• Vibration isolation: floating room, floating table.
• Temperature: At present we have tested temperatures from 1.5K up to 22K. We are in the process of implementing a ³He system, which will run down to 500 mK in continuous operation. Our STM can track the same atomically resolved locations throughout the temperature range.
• Magnetic field: 9 Tesla vertical, and 3 Tesla rotatable in-plane. Our STM can track the same atomically resolved locations as the field is swept over the full range.

Vortex Imaging in Iron Pnictide Superconductor Ba(Co_xFe_1-x)₂As₂

The discovery of iron-arsenic based superconductors (pnictides) in early 2008 broke the monopoly that cuprates had held over high-temperature superconductivity for more than two decades and led to new hope that the study of pnictides as a foil for cuprates could lead to breakthroughs in the understanding of high temperature superconductors. Additionally, the pnictides are more isotropic in a magnetic field, which may facilitate application due to more effective pinning of quantized magnetic vortices whose motion causes dissipation.

We carried out a study of optimally doped BaFe_1.8Co_0.2As₂ single crystals. The Co dopants reside in the more strongly bound Fe-As layer; therefore the cleaved surface retains the same doping as in the bulk. The Ba atoms remaining on the cleaved surface arrange into a stripelike 2×1 pattern. An atomic resolution topographic image is shown in Figs. 2a,b.

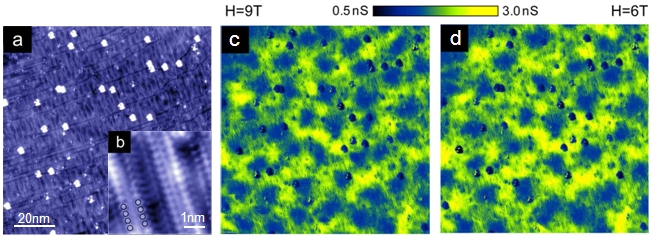
Fig. 2 STM images of BaFe_1.8Co_0.2As₂. (a) 100 nm × 100 nm topography, showing single-atom impurities as bright white spots. (b) Magnified inset shows atomic resolution with 2×1 surface reconstruction. (c) and (d) dI/dV images at the coherence peak energy E=5meV, show vortices as broad blue regions of depressed conductance, at 9 Tesla and 6 Tesla, respectively.

We used spectroscopic imaging to study the magnetic vortices in this material. At 9T and 6T we imaged vortices electronically by mapping the conductance (proportional to the density of states) at an energy where a vortex destroys the coherence peak of the superconducting gap. Figs. 2c,d show differential conductance maps at E=5meV, where vortices are visible as dark features due to the local suppression of the density of states at this energy. The vortices form a disordered lattice, consistent with isotropic, single flux quantum vortices.

Much can be learned about vortex interactions and pinning by studying the locations of vortices with respect to each other and to other structures within the superconductor. Vortex interactions can be roughly categorized into three scenarios. In the absence of strong pinning sites, vortices crystallize into a hexagonal lattice, shown in schematically in Fig. 3a. In the presence of strong pinning sites, the vortex arrangement with respect to those sites depends on the anisotropy of the material. In highly anisotropic superconductors, a one-dimensional vortex line may split like a stack of pancakes into point-like objects with the freedom to move independently in each superconducting layer. In this scenario, pancakes may find pinning sites independently in each layer, resulting in a high correlation between observed vortex and pinning locations in any given layer. This scenario is shown schematically in Fig. 3b. Finally, in a more isotropic superconductor with strong pinning sites, the vortices must remain as line objects, and can bend only slightly between layers to maximize their overlap with impurities throughout the bulk. In this scenario, there may be very little observable correlation between vortex locations and impurities in any one layer. This third scenario is depicted schematically in Fig. 3c. As shown in our data in Figs. 2c,d the vortices in BaFe_1.8Co_0.2As₂ form a disordered lattice, indicating strong pinning. There is no correlation between the locations of vortices and surface-impurities, proving that bulk pinning, and not surface pinning, must play a significant role in this superconductor. This third scenario is the most promising for technological applications because vortex motion causes dissipation and if vortices are pinned through several layers by a single pinning site it is far easier to engineer sufficient pinning sites.

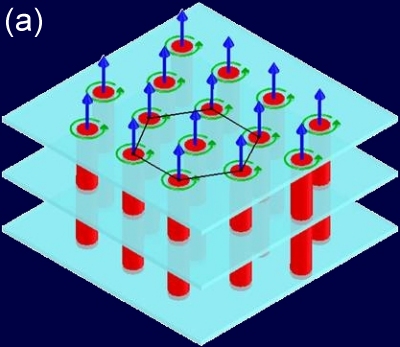
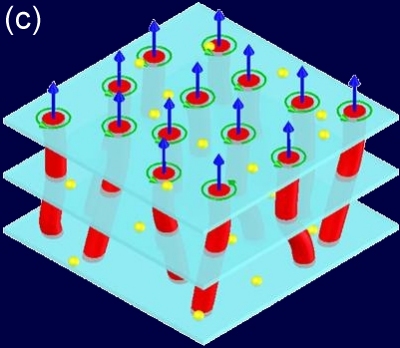
Fig. 3 Schematic illustrations of 3 different vortex pinning scenarios. (a) Negligible pinning; vortices form a lattice. (b) Strong pinning, large anisotropy; vortices separate into pancakes connected by Josephson vortices between layers. (c) Strong pinning, small anisotropy; vortices form a disordered lattice as they bend slightly to accommodate bulk pinning sites.

Collaborators

Xianhui Chen, University of Science & Technology, China

Funding

NSF DMR-0508812, AFOSR FA9550-05-1-0371

Vortex Liquid in Cuprate Superconductor Bi₂Sr₂CuO_6+x

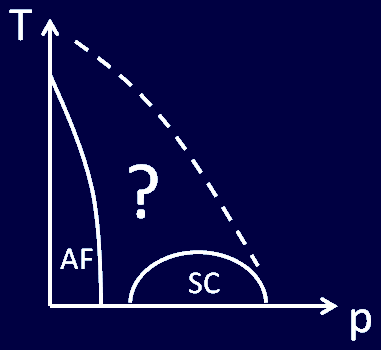
Fig. 4 Cuprate phase diagram: Temperature vs. doping.

The phase diagram of the cuprate superconductors contains, in addition to the antiferromagnetic and superconducting phases, a mysterious phase called the pseudogap (shown under the dashed line in the phase diagram in Fig. 4). In the pseudogap regime the density of states (DOS) is depressed at the Fermi level but transport measurements do not show bulk superconductivity. The apparent smooth evolution of the pseudogap down through the transition temperature into the superconducting gap has led some to argue that the pseudogap is a true pairing gap, a precursor to phase coherent superconductivity. Others have argued that the pseudogap is a signature of a competing or irrelevant phase. In either case, an understanding of the pseudogap will likely be a necessary precursor to a full theoretical description of cuprate superconductivity.

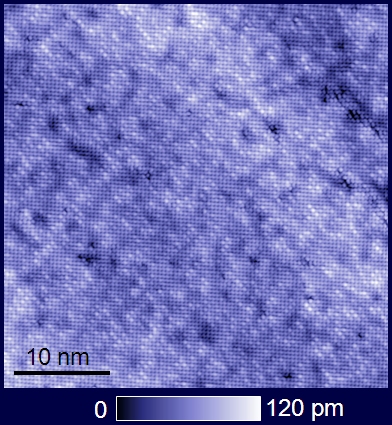
Fig. 5 Atomic resolution topography of the surface of (Bi_1-yPb_y)₂Sr₂CuO_6+x. (Pb doping is used to remove the supermodulation, but has no apparent effect on the density of state.)

Overdoped Bi₂Sr₂CuO_6+x is a good material for studying the pseudogap phase. This cuprate is a layered material which cleaves on an atomically flat surface, exposing a clean and stable surface that is well-suited for STM studies, as shown in Fig. 5. The low superconducting T_c of 15K allows easy comparison of states inside and outside of the superconducting dome with minimal thermal broadening. The upper critical field H_c2 is also accessible, approximately 9 Tesla at liquid helium temperature.

In the Hudson lab's prior work [1] the temperature was tuned to compare the DOS in the superconducting dome to that directly above it in the pseudogap phase, symbolized by the red arrow in the three-dimensional phase diagram in Fig. 6. In a complementary study, we have used our STM to compare the DOS in the superconducting dome to the DOS at the same doping and temperature in magnetic fields up to 9T, essentially leaving the superconducting dome through a perpendicular path, as represented by the blue arrow. The critical factor in both of these studies is the ability to compare spectra at exactly the same spatial location as an external parameter is tuned. Cuprates exhibit an intrinsic nanoscale spatial inhomogeneity throughout the phase diagram. Maintaining spatial registry through changes in temperature or magnetic field is crucial in order to strip out the intrinsic inhomogeneity and clearly identify the changes that occur on crossing the superconducting T_c or H_c2.

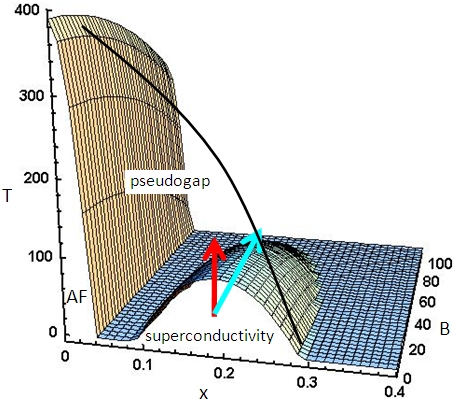
Fig. 6 3-dimensional phase diagram of cuprate superconductors, as a function of doping, temperature, and magnetic field.

At a temperature of 6 K, we find the gap depth from the spatially averaged dI/dV spectrum decreases with increasing magnetic field, which indicates a vortex liquid state. By tracking atomically resolved locations at different magnetic fields, we apply a normalization technique to remove inhomogeneities in the underlying density of states, revealing a more homogeneous superconducting state.

[1] M. C. Boyer, W. D. Wise, Kamalesh Chatterjee, Ming Yi, Takeshi Kondo, T. Takeuchi, H. Ikuta, E. W. Hudson, "Imaging the two gaps of the high-temperature superconductor Bi₂Sr₂CuO_6+x". Nature Physics 3, 802 (2007). (link)

Collaborators

Hiroshi Ikuta, Nagoya University

Funding

NSF DMR-0508812, AFOSR FA9550-05-1-0371

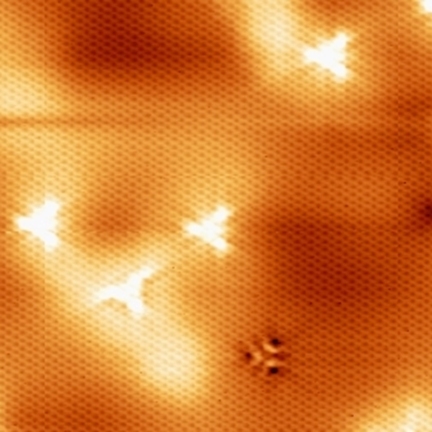
Fig. 7 An atomically resolved STM topography (20 nm × 20 nm) of doped Bi₂Se₃, showing a hexagonal lattice and triangular impurities (Se vacancies) at different heights.

Topological Insulators

Bi₂Se₃, with a large band-gap and simple bandstructure is expected to be the most useful of the known topological insulator materials. We are studying the novel properties of these topologically protected surface states.

Collaborators

Young Lee, MIT
Yoichi Ando, Osaka University